Tag: science

Aug 22 2010

More colours than the rainbow

500px-CIE1931xy_blank.svg
This post is about making the bridge between how a physicist understands colour, and something a bit more useful.

Light is a collection of electromagnetic waves; for a physicist the most important property of a wave is its wavelength, its “size”. The wavelengths of visible light fall roughly in the range 1/1000 of a millimetre to 1/2000 of a millimetre. (1/1000 of a millimetre is a micron). Blue light has a shorter wavelength than red light.

Things have colour either because they generate light or because of the way they interact with light that falls upon them. The light we see is made of many different wavelengths, the visible spectrum. Each wavelength has a colour, and the colour we perceive is a result of adding all of these colours together.

The diagram to the right summarises this: it’s called a chromaticity diagram, the numbers around the edge are wavelengths in nanometres (a millionths of a millimetre), pure, single wavelength light falls on this line; any point inside the line is formed from the mixture of wavelengths. The line represents “all the colours of the rainbow”; colours inside the line are not in the rainbow. The chromaticity diagram is the “periodic table” for colour scientists, it’s iconic and it summarises the world of colour.
This chromaticity diagram is just a slice through a volume, we could draw another one a little bit dimmer, and a little bit dimmer than that until we reached black.

How do we get to this diagram? The central issue to understanding perceived colour is that although the light in the environment comes as a mixture of a multitude of wavelengths, our eyes are limited by the light sensitive cells they contain, known as “cones”. In humans cones come in three types, which are sensitive in three different ranges of the spectrum. Roughly there are red-, blue- and green sensitive cones. So the eye gives just three readings in terms of colour description. The chromaticity diagram comes from a calculation trying to predict these three values and combining them to fit on a flat page (which only gives you two dimensions to play with).

Some other animals don’t have three sorts of cones. Birds, for example, have four – this is known as tetrachromacy which sounds to me like some sort of wizardry involving chairs (I’m reading Terry Pratchett at the moment). Birds have an extra type of cone in the ultra-violet part of the spectrum – so they are sensitive to wavelengths which we are not. Most mammals are dichromatic, but other primates, like humans, are also trichromatic. Dichromatic animals will be able to perceive a smaller range of colours than us. The evolutionary implication here is that earlier mammals lost some colour sensing ability, possibly for a gain in low light sensitivity but some mammals subsequently regained the ability.

The chromaticity diagram is still something of a physicists play-thing, it’s useful for doing calculations. There are other ways of describing colour which are related to human perception, they are developments based on the first steps used in constructing the chromaticity diagram. The aim of these methods is to similar numbers to colours that look similar; make those numbers reasonably easy to explain from a conceptual standpoint and try to give numbers twice as big to colours that are twice as bright. My favoured system in this respect is the CIE LAB system. The colours are expressed as three numbers L, a and b: L tells you something about overall brightness, a tells you the point on a scale between red and green and b tells you a point on the scale between yellow and blue.

But all of this is a bit of a fraud, because actually the colours you’re seeing on your monitor aren’t the real colours I’m trying to show you. The problem is that display devices contain red, green and blue elements but they don’t fall anywhere near the extremes of the chromaticity diagram and we can only get colours inside the the triangle defined by the red, green and blue elements in the monitor. A typical monitor gamut is shown here.

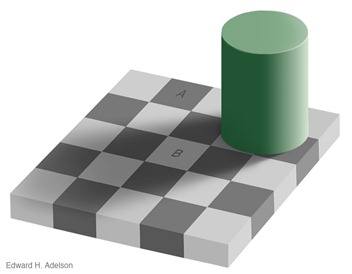
All this is based on the study of ideal colour stimuli (little square patches) on grey backgrounds, things get an awful lot more complicated if we start to worry about context. This is best illustrated with an image:

Adelson

As far as my computer is concerned squares A and B have the same colour, my brain and your brain are interpreting the scene context and giving them different colours. This is called Adelson’s checker shadow illusion.

Aug 22 2010

“Intimidating equations”

Taking lessons from Goldacregate, I’ve removed all the rant and sarcasm from this post.
In this article in today’s Observer, we’re advised that this:
PA = gUG + min(k – g, (1 – g)(1 – r))
is an “intimidating” equation”. Only if we’re easily scared! It relates the profit gained from dynamically priced airline tickets to some variables. This equation really is a very straightforward it says:
“profit equals two things multiplied together plus the smallest of two other things”
Using a Greek letter (capital pi) with a superscript following is a bit of showmanship, P would have done perfectly well in this instance. You can read the paper from which it is drawn here. It is written in the style of a paper in pure mathematics, which might explain the intimidation of the journalists in question.
I wrote a little bit about maths a while back: maths is the language of much of the science I do, but its a convenient tool – it’s not an end in itself. The seed of “Goldacregate” was a query by a journalist as to how to read out an equation, the thing is that practitioners rarely speak equations out loud: they scribble them on the nearest available surface (often illegibly, and incorrectly) or fight endless battles with machines to get them into electronic documents. Furthermore there is a long and dishonourable history of public relations companies using essentially meaningless equations to promote products and services.
For non-users of equations they are simply a cloak, a cloud of chaff thrown up to hide the truth beneath. For users, they are a compact and exact way of writing down the truth.
The next time you see an equation, don’t be scared beneath it there is something simple which can be said.
Unexpurgated version: Ah, bless, the economists are playing at being scientists by using an equation and the journalists have got the vapours at the impossible complexity of it all. Nasty equation: please, don’t hurt me.

Jul 28 2010

Spider silk

Photo by Fir0002/flagstaffotos (GFDL license)

I’ve never worked on spider silk myself, but my work on synthetic polymers and biological physics took me to conferences where spider silk work was presented and it always struck me as a very interesting. Spider silk has a rather impressive set of material properties, yet it is produced rapidly at the back end of a spider under everyday conditions. This is a pretty electron micrograph of spider spinnarets from where the silk comes (warning: page includes creepy crawlies).

I introduced molecules, and proteins back in this post. Proteins are the key molecules used to make organisms, an organism’s DNA are the instructions to make a set of proteins. Spider silk is made from protein. A spider is able to produce a whole range of silks with different physical properties: dragline silk is used to make the outer-rim and spokes of a web and is strong and tough; capture-spiral silk is sticky, stretchy and tough; tubiliform silk is used for egg cases and is the stiffest; aciniform silk used for wrapping prey is the toughest; minor-ampullate silk used to make temporary scaffolding for building a web (it’s not as strong but very stretchy). From a technical point of view “strong” refers to how hard it is to stretch something, and “tough” refers to how hard it is to break something. Spider silk is similar to silkworm silk but it is stronger and more extensible.

The properties of spider silk arise from it’s microstructure, essentially the protein molecules make a very fine net held together with little crystals. The fact that crystals form is a function of the protein structure, exactly how many and what distribution of crystals form is influenced by how the spider treats the silk-protein solution as it comes out of it’s spinnarets. Precisely how the spider achieves this isn’t entirely clear, the protein starts off in a liquid solution, the spinnarets force the liquid out into the air whilst changing things such as the salinity, concentration and pH of the liquid and “Hey, presto” it turns into silk! It would be nice if we could farm spiders for their silk unfortunately this is difficult, they just don’t get on with each other.
The strength of natural materials is often compared to that of steel, but there is a trick to watch out for here: the comparison is often based on weight. Steel is about x10 denser than silk, so your strand of equivalent strength is rather fatter if it is made from silk.

The closest synthetic material to spider silk in terms of it’s strength per weight is Kevlar. Kevlar is processed using hot sulphuric acid under high pressure which as you might imagine is not very nice. Spider silk, on the other hand is made at room temperature and pressure from an aqueous solution of benign materials.  Not only this, a spider can eat the silk it’s already made and use it to make more silk. As scientists, this makes us more than a little bit jealous.

Not only is spider silk interesting of itself, but from a material scientist point of view, it really isn’t fun to make and use new polymers (you need to build expensive plant to make them, you need to work out your ingredient supply chain, you need to check for safety and environmental problems). If, on the other hand, you can get the properties you want from one of your pre-existing polymers by changing the microstructure then life is much easier. Spider silk may provide hints as to how this might be done.

The neat thing about this story is that it illustrates an important point: we can genetically engineer bacteria and goats to produce the protein in spider silk but not make nice silk-like stuff. Knowing the sequence of amino acids that a spider is making is not enough to make silk. In much the same way knowing the proteins that go up to make up a human is rarely enough to understand, let alone cure, a disease. 

Scientists have done research on the effect of different drugs on web spinning, filmmakers have made some fun of this experiment* (warning: contains spiders). Other interesting biomaterials include, mollusc adhesive and slug slime and I’ve already written about why butterflies are blue.

Update: Curtesy of @happymouffetard, the evolutionary origin of spider-silk spinnarets appears to be hair follicles, according to this article.


*Thanks to Stephen Curry for pointing me to the “spiders on drugs” video.

Jul 22 2010

The Periodic Table

Understanding the Periodic Table is very much like making love to a beautiful woman, there’s no point rote-learning the location of the different elements if you don’t know what they do… langtry_girl*

The Periodic Table of the Elements is a presentation of the known elements which provides information on the relationships between those elements in terms of their chemical and physical properties. An element is a type of atom: iron, helium, sulphur, aluminium are all examples of elements. Elements cannot be broken down chemically into other elements, but elements can change. An atom is comprised of electrons, protons and neutrons.

This is all very nice, but if you look around you: at the wallpaper, the computer screen, the table – very little of what you see is made from pure elements. They’re made from molecules (pure elements joined together), and the molecules are arranged in different ways which may be completely invisible. So in a sense the periodic table represents the bottom of the tree of knowledge for people interested in materials, other scientists may be more interested in what makes up the elements.

The periodic table, approximately as it is seen today, was discovered by Dmitri Mendeleev in 1869, he designed it based on the properties of the elements known at that time. For a scientist the Periodic Table is pleasing, it says of the elements: “this many and no more”. It also stands as one of the great scientific predictions: Mendeleev proposed new elements based on his table constructed from the known elements and ,behold, they appeared with roughly the properties he expected.
Mendeleev’s periodic table was a work of organisation, it later turned out through the discovery of quantum mechanics that the periodicity and order found in the table can be derived from the behaviour of electrons in atoms.
To reverse a little, there is scope for more elements in the periodic table, they appear tacked on at the end of the table and are made artificially. The experimental scheme to achieve this is to fire atoms of existing elements into each other in the in the hope that they’ll fuse, occasionally they do, but the resulting atoms have a fleeting existence. They are rarely found in any number and vanish in fractions of a second, they are not elements of which you can grab hold. This has always struck me as being akin to flinging the components of a car off a cliff and claiming you have made a car when momentarily the pieces look like a car as they plummet to the ground.
I had a struggle here deciding whether to describe the periodic table as being designed, invented, or discovered. I stuck with discovered, because discovering is what scientists do, inventing is for inventors and designing is for designers ;-) It does raise an interesting philosophical question which has no doubt been repeatedly discussed down through the ages.

As a design, shown above, the periodic table is a cultural icon which everyone knows. Even if they don’t understand what it means, they know what it stands for – it stands for science. How to make sure people know your scene is set in a lab or your character is a scientist? Bung in a periodic table. It has been purloined to organise other sorts of information, such as Crispian Jago’s rather fine “Periodic Table of Irrational Nonsense“, some more examples here. There is a song.

At various times in my life I’ve been able to name and correctly locate all the elements in the periodic table, normally takes a bit of effort and some mnemonics to help. Increasingly now, I can remember the mnemonics but not the elements they refer to.

Different parts of the periodic table are important to different sorts of scientists. To organic chemists carbon (C), hydrogen (H), oxygen (O), nitrogen (N) hold the majority of their interest with walk on parts for some of the transition metals (the pink ones in a block in the middle) which act as catalysts. Inorganic chemists are more wide ranging, only really forbidden from the Noble Gases (helium (He), neon(Ne), argon (Ar), krypton (Kr), xenon (Xe)) which refuse to react with anything. Semi-conductor physicists are after the odd “semi-metals”: silicon (Si), indium (In), gallium (Ga), germanium (Ge), arsenic (As). For magnets there’s iron (Fe), cobalt (Co), nickel (Ni) along with other transition metals and the Lanthanides. The actinides are for nuclear physicists, radiation scientists and atomic bomb makers. Hydrogen is for cosmologists. In this view, as a soft condensed matter physicist, I am closest to the organic chemists.

I’m rather fond the periodic table, it is the scientist’s badge, but I’m scared of fluorine.

*To be fair to langtry_girl, I pondered on twitter “Trying to finish the sentence: “Understanding the Periodic Table is very much like making love to a beautiful woman…” and I think hers was the best reply. It is, of course, a reference to Swiss Toni.

Jul 12 2010

How does a magnet work?

How does a magnet work? This question arose on “I’m a Scientist, Get me out of here“, a fine piece of science communication which involved putting scientists in contact with school children. This is my attempt at an answer, which says a bit more about science in general but is utterly untimely. The short answer to the question is that magnets are made from atoms which act like little magnets and in a proper magnet are all lined up, but as an answer this is somewhat unsatisfactory.

From a scientific point of view, what you’d commonly call magnets are just one group of magnetic materials – the ferromagnets. They are accompanied in early magnetism courses for aspiring physics students by paramagnetic and diamagnetic materials. A ferromagnetic material, like iron, is strongly attracted to a magnet, a paramagnetic material is weakly attracted and a diamagnetic material is very weakly repelled. Diamagnetism and paramagnetism are useful for scientific research but it is ferromagnetism where all the practical applications are found. Iron, cobolt and nickel are the only ferromagnetic elements.

At this point I am ashamed to admit I nearly missed out on a tortured analogy to explain magnetism but fortunately I caught myself in time! Imagine, if you will, a crowd bearing vuvuzelas. Individuals in this crowd can blow their vuvuzelas in any direction they please, however much we might wish they didn’t. In a ferromagnet groups of vuvuzela players spotting their neighbours spontaneously face the same direction to play their devilish instruments. The whole crowd may not be blowing them in the same direction but groups of them will. They can be marshalled to all blow their horns in the same direction by a band leader, and once pointing in the same direction they will continue to face that way, even in the absence of the band leader.

The individuals in this group are atoms in a material, and the vuvuzelas represent the magnetic field of a single atom. Groups of players facing in the same direction represent magnetic domains and the band leader represents an applied magnetic field. The point about ferromagnets is they massively enhance an a magnetic field applied by something like a coil of wire with a current flowing through it – this is how you make an electromagnet. The difference between a “magnet” and any old bit of ferromagnet is that in a “magnet” all the domains have been lined up to face the same way.

In paramagnetic materials vuvuzelas players ignore their neighbours and play away in random directions, they respond in a somewhat feeble fashion to the directions of the band leader.

In diamagnetic materials the crowd have no vuvuzelas but use their hands as a substitute, rather petulantly they face the opposite direction to that proposed by the band leader. In scientific language the hands represent induced magnetic dipole moments.

But why is an atom magnetic? An atom could be magnetic because the electron orbiting the nucleus acts like a little current loop, which gives it a magnetic field like a little bar magnet (posh name for “little bar magnet” is “magnetic dipole moment”) but actually the majority of the magnetic dipole moment of an atom comes from the intrinsic magnetic dipole moment of individual electrons.

We really don’t know why the electron acts as a magnetic dipole, it is ascribed to a property known as ‘spin’ but it can’t be spin as we normally define it since electrons are, as far as we can tell, point-like – nobody has every managed to measure the diameter of an electron. Therefore how can we meaningfully describe it as spinning? In a sense the origin of the electron magnetic dipole moment is not important, it exists, we know what it is and we can use the measured value in our calculations for designing magnetic materials. This question of the “why” of fundamental properties of sub-atomic particles is what string theory seeks to address. For most scientists the answer is unimportant for practical applications, but for physicists in particular it is a nagging unpleasantness that we don’t know why.

Magnetism and electricity have been known since antiquity but as two very separate phenomena, and unsurprisingly really. Magnetism is a property of some funny rocks (lodestone) whilst electricity is a property of rubbed materials, and lemons. The connection between the two is far from obvious, the link was made in the early part of the 19th century. This is a recurring theme in science, we blithely teach that such and such is true, implying that it’s truth is pretty much self-evident and elide the fact that for most of history we have not believed these things and that it has taken the painstaking work of a number of very great minds to reveal these self-evident truths. I must admit to being a little unsure of the history in this area, taught in England the key figures were Michael Faraday who did much of the experimental work in linking electricity and magnetism followed by James Clerk Maxwell who formulated a mathematical theory. In the experimental area in particular there were many other participants in the story, I suspect who takes centre stage depends on where you are taught.

So there you have it: magnets work because the vuvuzela players copy each other and play in the same direction.